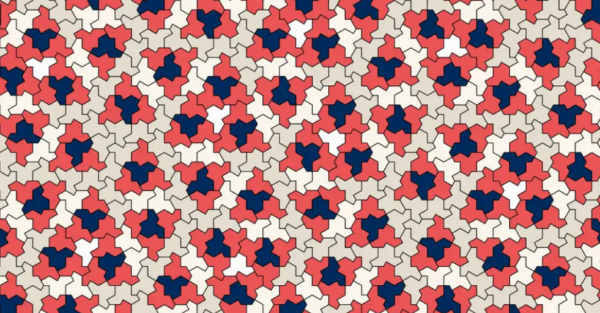
A brilliant team has just solved a very difficult tile-related puzzle. The researchers discovered a previously undiscovered shape: a 13-sided structure termed “the hat” that can tile a surface without repeating itself.
The hat is an aperiodic monotile, which implies that a single form may cover a whole surface without any translational symmetry or ever repeating pattern. Penrose tilings are an example of aperiodic tiling in which the pattern is aperiodic yet employs two distinct forms.
Eight polykite kites form the 13-sided hat.

The tiling of the hat consists of only one shape, a “einstein” (German for “one stone”), making the design an aperiodic monotile. The 13-sided hat is a polykite comprised of eight linked kites. In a preprint document published online this month, a research team led by the mathematician David Smith and his colleagues demonstrated the existence of an aperiodic monotile.
“You are essentially searching for a one-in-a-million occurrence. Chaim Goodman-Strauss, a mathematician at the National Museum of Mathematics and co-author of the study, told New Scientist, “You filter out the 999,999 uninteresting ones, and you’re left with something strange, which is worth further examination.” “And then by hand you begin scrutinizing them, attempting to comprehend them, and removing their structure. In this case, a computer would be useless since a person must build a proof that a human can comprehend.”
The discovery appears to settle a long-standing conundrum in the discipline of geometry, according to mathematicians. Yet, for the rest of us, it may provide an innovative new alternative for bathroom tiling.